As the study on the 3D quantum Hall effect marches forward, physicists feel puzzled by the new findings. Recently, SUSTech research team proposed a new theory to answer the questions.   

The team was led by Professor Haizhou Lu of the Department of Physics and Shenzhen Institute for Quantum Science and Engineering at SUSTech. Their work, entitled “Theory for the charge-density-wave mechanism of 3D quantum Hall effect” was published in Physical Review Letters [1] The article is selected as the Editors’ selection and recommend as a Viewpoint in Physics by the American Physical Society.

In 1980, the discovery of the quantum Hall effect in the semiconductor of the two-dimensional electron gases opened one of the most exciting chapters in physics history. At very low temperatures and in very high magnetic fields, Hall resistance is quantized into an integer multiple of h/e2. This is called the quantum Hall effect (h is Planck’s constant and e is the electronic charge)[2]. Since then, physicists have discovered various versions of the quantum Hall effects, such as the quantum anomalous Hall effect where the magnetic field is not needed [3]. Three Nobel Prizes came to birth because of the quantum Hall effect. Related topological states of matter have also become an important research field.

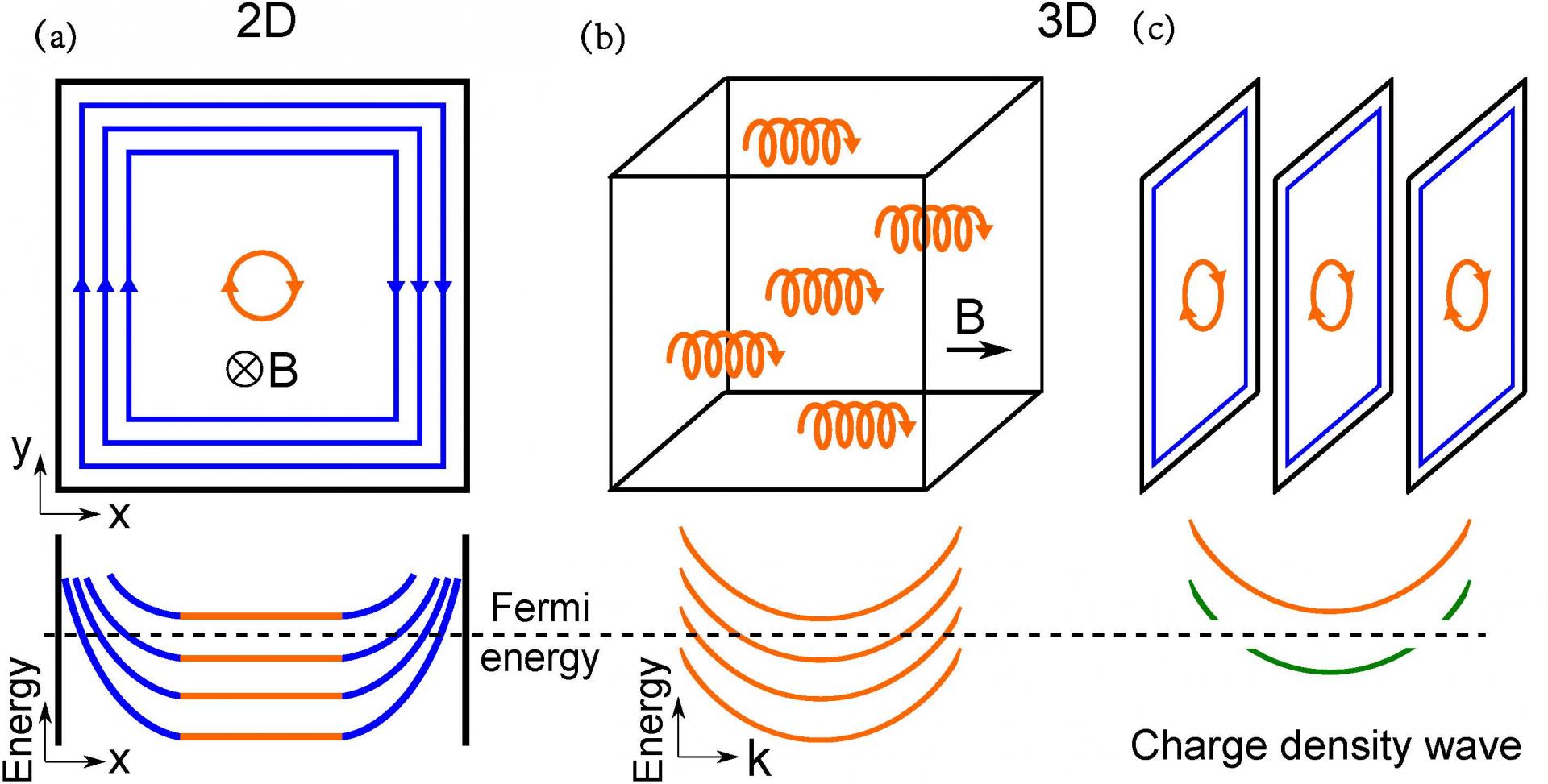
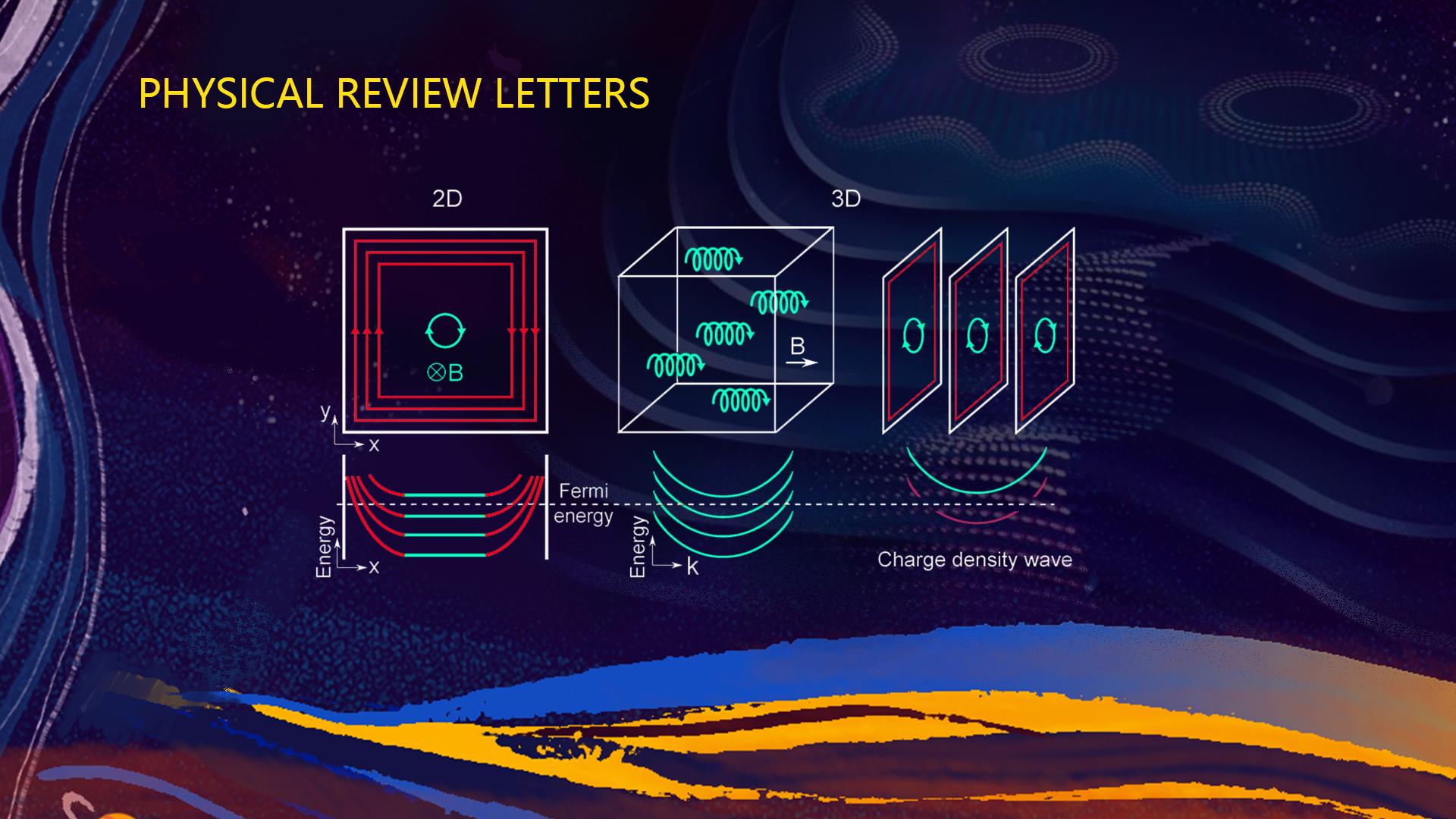
Left figure: In two dimensions, the quantum Hall effect arises when only the edge states (blue) conduct electrons, while the interior bulk states are insulating as the Fermi energy lies between the Landau levels. Center figure: In three dimensions, the Landau levels turn to one-dimensional bands of Landau levels that disperse with the momentum (k) along the direction of the magnetic field. The quantum Hall effect is difficult in three dimensions because the bulk is metallic as the Fermi energy always crosses some Landau bands. Right figure: The charge density wave may gap the Landau band so that the bulk is insulating and the quantum Hall effect can be observed. These figures are from Ref. [1].

It used to be difficult to realize the quantum Hall effect in a 3D system. Physicists had been looking for a 3D version of the quantum Hall effect for over 30 years [4-7] but failed to get convincing results. In 2019, Prof. Liyuan Zhang’s group from SUSTech successfully observed a 3D quantum Hall effect in ZrTe5 [8]. Using high-quality samples with high mobility and low electron concentration, they observed quantized Hall resistance platforms and vanishing longitudinal resistivity in the magnetic fields ranging from 1.7 to 2.1 Tesla at 0.6 Kelvin. In that experiment, the charge density wave, a correlated phase that can divide a three-dimensional system into layers of 2D quantum Hall effects (see the figure), is considered as the most desirable mechanism. 

Physicists felt puzzled about these new results: What are the quantitative physical mechanisms behind the observed three-dimensional quantum Hall effect? Why does the Hall platform exist only within a certain range of the magnetic field? The theory proposed by Hai-Zhou Lu’s group, also from SUSTech, solves these two problems [1]. In their model, a magnetic field-induced charge density wave can be used to explain the 3D quantum Hall plateau. They found that, with an increasing magnetic field, electron-phonon interactions can induce a second-order phase transition to the charge density wave. They also found that there are both commensurate and incommensurate charge density waves, and the ground-state energy of the commensurate charge density wave is lower between 1.7-2.1 Tesla, thus explaining the Hall plateau of the magnetic field in this segment and the absence of the Hall resistance plateau above 2.1 Tesla.

In the last century, physicists understood the world mostly in terms of the phase transitions with symmetry breaking described by order parameters, while in this century, topological phase transitions have gradually become the new insights to explore new physics. This paper theoretically explores a rare case connecting two centuries, in which the same magnetic field induces an order phase transition in one direction but topological phase transition in the other two directions. This will lead to more exciting discoveries.

The first author of the work is Dr. Fang Qin (the SUSTech Presidential Postdoctoral Fellowship) and the corresponding author is Hai-Zhou Lu. The co-authors include Professor Wenqing Zhang (Department of Physics, SUSTech), Academician Dapeng Yu (Shenzhen Institute for Quantum Science and Engineering and Department of Physics, SUSTech), and Academician X. C. Xie (Peking University).

This work was supported by the National Natural Science Foundation of China, the Strategic Priority Research Program of Chinese Academy of Sciences, Guangdong province, the National Key R&D Program, the Natural Science Foundation of Shanghai, Shenzhen High-level Special Fund, and the Science, Technology, and Innovation Commission of Shenzhen Municipality, China Postdoctoral Science Foundation, the SUSTech Presidential Postdoctoral Fellowship, and Center for Computational Science and Engineering of Southern University of Science and Technology.

Paper link: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.125.206601

References

[1] F. Qin, S. Li, Z. Z. Du, C. M. Wang, W. Q. Zhang, D. P. Yu, H. Z. Lu, and X. C. Xie, “Theory for the charge-density-wave mechanism of 3D quantum Hall effect”, Phys. Rev. Lett. 125, 206601 (2020).

[2] K. von Klitzing, et al., “New method for high-accuracy determination of the fine-structure constant based on quantized Hall resistance”, Phys. Rev, Lett. 45, 494 (1980).

[3] C. Z. Chang, J. Zhang, X. Feng, J. Shen, Z. Zhang, M. Guo, K. Li, Y. Ou, P. Wei, L. L. Wang, Z. Q. Ji, Y. Feng, S. Ji, X. Chen, J. Jia, X. Dai, Z. Fang, S. C. Zhang, K. He, Y. Wang, L. Lu, X. C. Ma, and Q. K. Xue, “Experimental Observation of the Quantum Anomalous Hall Effect in a Magnetic Topological Insulator”, Science 340, 167 (2013).

[4] J. R. K. Cooper, et al., “Quantized Hall effect and a new field-induced phase transition in the organic superconductor (TMTSF)2(PF)6”, Phys. Rev. Lett. 63, 1984 (1989).

[5] S. T. Hannahs, et al., “Quantum Hall effect in a bulk crystal”, Phys. Rev. Lett. 63, 1988 (1989).

[6] S. Hill, et al., “Bulk quantum Hall effect in η-Mo4O11”, Phys. Rev. B 58, 10778 (1998).

[7] H. Cao, et al., “Quantized Hall effect and Shubnikov-de Haas oscillations in highly doped Bi2Se3: Evidence for layered transport of bulk carriers”, Phys. Rev. Lett. 108, 216803 (2012).

[8] F. Tang, Y. Ren, P. Wang, R. Zhong, J. Schneeloch, S. A. Yang, K. Yang, P. A. Lee, G. Gu, Z. Qiao, and L. Zhang, “Three-dimensional quantum Hall effect and metal-insulator transition in ZrTe5”, Nature 569, 537 (2019).